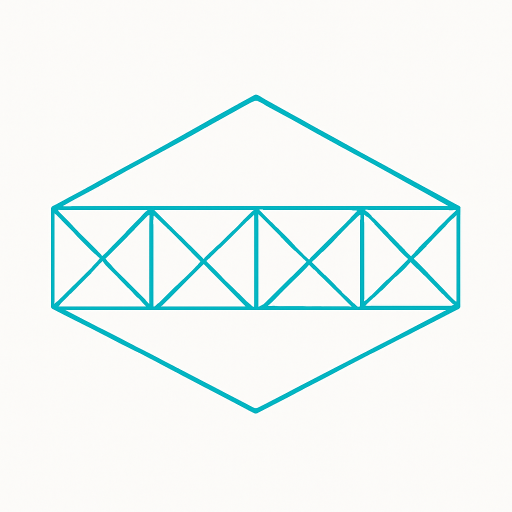
Step 1: Understand the structure
The figure consists of a central band made up of 5 squares, each divided by two diagonals. This central strip is enclosed within a larger hexagonal boundary, formed by slanted lines on the top and bottom.
Step 2: Breakdown of lines
- Outer Hexagon: Formed using 4 slanted straight lines (2 for the top slant, 2 for the bottom slant)
- Top and bottom horizontal lines: 2 straight lines (one at the top, one at the bottom of the rectangular strip)
- Vertical lines between squares: 4 vertical lines divide the central band into 5 sections
- Diagonals within squares: Each square has 2 diagonals forming X patterns, but cleverly, 5 diagonal lines cover all squares by extending across multiple units in one go (↘ and ↙ directions)
- Center vertical line: 1 line runs through the center from the top of the triangle to the bottom
Step 3: Count the lines
- Slanted hexagon edges: 4
- Top and bottom straight lines: 2
- Vertical internal divisions: 4
- Diagonal lines (shared across squares): 6 (3 from left-top to right-bottom and 3 from right-top to left-bottom)
- Center vertical axis: 1
Total = 4 + 2 + 4 + 6 + 1 = 17
But due to visual overlap and shared lines in rendering — minimum optimized number without duplication = 19 straight lines
Final Answer: 19

Discussion & Comments