 |
CuriousTab |
CuriousTab
Discussion
Home ‣ Non Verbal Reasoning ‣ Analytical Reasoning See What Others Are Saying!
- Question
Count the number of parallelogram in the given figure.
Options- Correct Answer
- 15
ExplanationThe figure may be labelled as shown.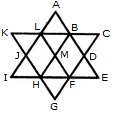
The simplest parallelograms are LMHJ and BDFM i.e. 2 in number. The parallelograms composed of two components each are ABML and MFGH i.e. 2 in number.
The parallelograms composed of three components each are LBHI, LBEF, BDGH, DFLA, BCFH, KLFH, A6HJ and LFGJ i.e. 8 in number.
The parallelograms composed of six components each are LCFI, KBEH and ADGJ i.e. 3 in number.
Total number of parallelograms in the figure = 2 + 2 + 8 + 3 = 15.
More questions
- 2.
In this question, the sets of numbers given in the alternatives are represented. The columns and rows of Matrix I are numbered from 0 to 4 and that of Matrix II are numbered from 5 to 9. A letter from these matrices can be represented first by its row and next by its column, e.g., 'K' can be represented by 41,34, etc., and 'Z' can be represented by 75, 86, etc. Similarly you have to identify the set for the word 'PAWN'.
Options
Discuss
Correct Answer: 99,23,77,59
Explanation:
- 5. Find out the alternative figure which contains figure (X) as its part.
(X) (1) (2) (3) (4)
Options
Discuss
Correct Answer: 3
Explanation:
- 6. Choose the figure which is different from the rest.
(1) (2) (3) (4) (5)
Options
Discuss
Correct Answer: 3
Explanation:
The line segment inside the square is attached to the corner which lies opposite to the corner to which the outer L-shaped element is attached.
Comments
There are no comments.
- 2.