Find the number of triangles in the given figure?

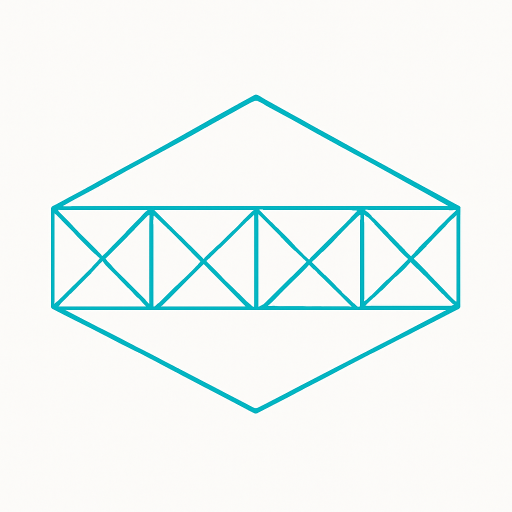
Question: Find the number of triangles in the given figure.

Step 1: Analyze the structure

The figure shown is a combination of a large triangle on top and a rectangular base, both of which are subdivided into smaller triangles using vertical, horizontal, and diagonal lines.

To find the total number of triangles, we need to count:

• Smallest individual triangles
• Medium-sized triangles formed by combining two or more small triangles
• Large triangles formed by combining multiple units

Step 2: Count systematically

• Top triangle: Contains 4 small triangles
• Middle row: 4 medium-sized triangles formed around the center
• Lower rectangle: 8 small triangles plus combinations forming medium and large triangles
• Composite triangles: Formed by combining multiple sections — at least 5 larger triangles exist

Total triangles = 4 (top) + 4 (middle) + 8 (bottom) + 5 (combined) = 21

Final Answer: 21 triangles