Discussion
Home ‣ Non Verbal Reasoning ‣ Cubes and Dice See What Others Are Saying!
- Question
- How many of the cubes have at most faces painted?
Options- A. 339
- B. 244
- C. 342
- D. None of these
- Correct Answer
- 339
ExplanationOut of 6 faces of 5 faces are exposed and those were painted.
Number of vertices with three faces exposed (Painted) is 4
Number of vertices with 2 faces exposed (Painted) is 4
Number of vertices with 1 faces exposed (Painted) is 0
Number of vertices with 0 faces exposed (Painted) is 0
Number of sides with 2 sides exposed (Painted) is 8
Number of sides with 1 sides exposed (Painted) is 4
Number of sides with no sides exposed (Painted) is 0
From the above observation:
Number of cubes with 3 faces Painted is 4
Number of cubes with 2 faces Painted is given by sides which is exposed from two sides, out of 8 such edges 4 vertical edges will give us 6 cubes per edge and 4 edges from top surface will give us 5 such cubes from each edge and required number of cubes is 6 x 4 + 4 x 5 = 44.
Number of cubes with 1 face Painted is given by faces which is exposed from one sides four vertical faces will give us 6 x 5 = 30 cubes per face and top face will give us 5 x 5 = 25 and required number of cubes is 30 x 4 + 25 x 1 = 145
Number of cubes with 0 face Painted is given by difference between total number of cubes - number of cubes with at least 1 face painted = 343 - 4 - 44 - 145 = 150
In other words number of cubes with 0 painted is 6 x 5 x 5 = 150
From the above explanation number of the cubes with 0 faces painted is 150.
From the above explanation number of the cubes with at most 2 faces painted is 150 + 145 + 44 = 339.
Or else 343 - 4 = 339 - 1. NA
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 2. Find the missing figure in the series from the given answer figures:

Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 3. Which answer figure will complete the pattern in the question figure?
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 4. Which answer figure will complete the pattern in the question figure?
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 5. Among the four answer figures, which figure can be formed from the cut?pieces given below in the question figure?
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 6. Which answer figure will complete the series?:

Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 7. NA
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 8. Select a figure from the given four alternatives which fits exactly into Figure-X to form a complete square.
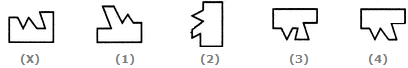
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 9. NA
Options- A. 1
- B. 2
- C. 3
- D. 4 Discuss
- 10.
In this question, the sets of numbers given in the alternatives are represented. The columns and rows of Matrix I are numbered from 0 to 4 and that of Matrix II are numbered from 5 to 9. A letter from these matrices can be represented first by its row and next by its column, e.g., 'K' can be represented by 41,34, etc., and 'Z' can be represented by 75, 86, etc. Similarly you have to identify the set for the word 'PAWN'.

Options- A. 44,10,87,55
- B. 99,23,77,59
- C. 03,40,86,65
- D. 31,31,59,66 Discuss
More questions
Correct Answer: 1
Explanation:
NA
Correct Answer: 1
Explanation:
On the basis of given figures in above question , we can see that
In each subsequent figure the design rotates through 90° anticlockwise.
Hence , figure ( 1 ) will come on the place of ? in question figure . As shown in answer figures .
Correct Answer: 1
Explanation:
NA
Correct Answer: 1
Explanation:
NA
Correct Answer: 2
Explanation:
Answer figure (2) can be formed from the cut-pieces given in the question figure.
Correct Answer: 3
Explanation:
As per the given figure in above question, it is clear that
In each subsequent figure the number of asterisks is increasing by one and the lower circle moves from left to right and vice-versa.
Clearly , figure ( 3 ) will come on the place of ? from answer figures .
Correct Answer: 1
Explanation:
Answer Figure (1) can be constructed from the parts given in question figure.
Correct Answer: 4
Explanation:

Correct Answer: 1
Explanation:
After opening the first fold it will look like as:
When it is unfolded completely it will look like as:
Correct Answer: 99,23,77,59
Explanation:
Comments
There are no comments.Programming
Copyright ©CuriousTab. All rights reserved.
