Correct Answer: 3
Explanation:
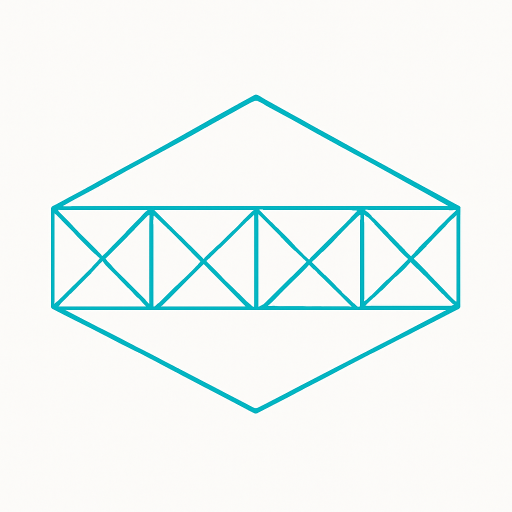
Question: What is the minimum number of colour pencils required to fill the spaces in the figure such that no two adjacent spaces have the same colour?
Step 1: Understand the problem
This is a classic graph colouring problem where each distinct region in a geometric figure must be coloured such that no two touching (adjacent) regions share the same colour.
Step 2: Analyze the figure
The figure appears to be a triangle divided into smaller regions — some triangular, some trapezoidal — all connected in a way that many regions share borders.
Step 3: Apply graph colouring logic
Step 4: Result
With smart arrangement and using non-touching colouring strategy, the puzzle can be solved using only:
3 colour pencils
Note: Although the figure looks complex, a well-structured colouring pattern ensures that no more than 3 colours are necessary to fill all adjacent areas without conflicts.


Discussion & Comments