Discussion
Home ‣ Verbal Reasoning ‣ Routes and Networks See What Others Are Saying!
- Question
- Which of the following is true?
Options- A. Statement 1 is true but statement 2 is false.
- B. Statement 1 is false but statement 2 is true.
- C. Statement 1 is true and so is statement 2.
- D. Statements 1 and 2 are false.
- Correct Answer
- Statement 1 is true and so is statement 2.
ExplanationAs per the given conditions,
we require surveillance cameras that would cover all roads converging at an intersection. The cost for covering all the roads has to be minimum.
If we put a camera on '1', roads from '7' and '6' to 1 will be covered.
If we put a camera on intersection '2', roads converging from '3' to '2' will be covered.
If we put a camera on '3', roads from '3' to '5', '6' to '5' and '7' to '5' will be covered.
Now we are left with roads from 7 to 4 and from 6 to 4 both of them converge from 4 hence these will be covered by putting a camera on '4'. And then all the roads will be covered and the minimum cost is 2 + 5 + 1 + 4 = 12 lakhs - 1. c _ bba _ cab _ ac _ ab _ ac
Options- A. abcbc
- B. acbcb
- C. babcc
- D. bcacb Discuss
- 2. NA
Options- A. 14th November
- B. 15th August
- C. 26th January
- D. 2nd October Discuss
- 3. Nebulous : Form
Options- A. Insincere : Misanthrope
- B. Benevolent : Excellence
- C. Insipid : Taste
- D. Composed : Innocence Discuss
- 4. 3 : 12 :: 5 :?
Options- A. 25
- B. 35
- C. 30
- D. 15 Discuss
- 5. Paper : Tree :: Glass :?
Options- A. Window
- B. Sand
- C. Stone
- D. Mirror Discuss
- 6. Statement:
Poverty persists under conditions where human resource is undervalued, and land and other material resources are overvalued.
Assumptions:
I. Poverty can be eradicated by enhancing the human resource value.
II. There should be a balance between the valuation of human and material resources.
Options- A. if only assumption I is implicit.
- B. if only assumption II is implicit.
- C. if either I or II is implicit.
- D. if neither I nor II is implicit. Discuss
- 7. 'Tall' is related to 'Dwarf' in the same way as 'Kind' is related to
Options- A. Weak
- B. Gentle
- C. Cruel
- D. Forgive Discuss
- 8. Truthfulness : Liar : : Loyalty :?
Options- A. Worker
- B. Traitor
- C. Diligent
- D. Faithful Discuss
- 9. Statements:
H * T, T S B, B © R
Conclusions:
I. R © H II. B © H III. T % R
Options- A. Only i is true
- B. Only I and II are true
- C. Only I and III are true
- D. Only II and III are true Discuss
- 10. _OPQ, N_PQ, NO_Q, NOP_
Options- A. ONQP
- B. NOQP
- C. NOPQ
- D. PQNO Discuss
More questions
Correct Answer: acbcb
Explanation:
The given series is in the below pattern , we can see that
cabbac / cabbac / cabbac.
Hence , required answer will be acbcb .
Correct Answer: 14th November
Explanation:
All others are national holidays.
Correct Answer: Insipid : Taste
Explanation:
A nebulous from means having an ambiguous from same as an insipid taste which is dull, boring, almost no taste.
Correct Answer: 30
Explanation:
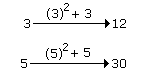
Correct Answer: Sand
Explanation:
As paper is product of tree. Similarly glass is product of sand.
Correct Answer:
Explanation:
if both I and II are implicit.
Correct Answer: Cruel
Explanation:
'Tall' is opposite to 'Dwarf' and 'Cruel' is opposite to kind.
Correct Answer: Traitor
Explanation:
Former cannot be expected from the latter.
Correct Answer:
Explanation:
H < T ...(i) T ? B ...(ii) B > R ...(iii)
From (i) and (ii) we get H < T ? B or B > H.
Hence III does not follow.
Correct Answer: NOPQ
Explanation:
Fill the blank space with correct alphabets as NOPQ word is repeating.
N O P Q , N O P Q, N O P Q, N O P Q ? NOPQ
Comments
There are no comments.More in Verbal Reasoning:
Programming
Copyright ©CuriousTab. All rights reserved.
